


The Descending Staircase
and the Idea of Strange Loops
Where Linear & Cyclical Meet
in One Composite Movement
The idea of a strange loop was first introduced by Douglas Hofstadter
in his remarkable book on language, computers and human perception,
Gödel, Escher, Bach (1979). I like to think of strange loops not so much
as a paradox of logic, however, but rather as a unique form of natural
movement, one which is at once both
linear and cyclical.
A strange loop is linear in the sense that a straight-line trajectory
of change is implied which, much like an arrow or a bullet, moves
out towards some distant target or horizon, and then
does not return;
At the same time, a strange loop is also cyclical. This is because
a strange loop also moves towards some distant goal and then
returns to its origin or point of departure.
This intermingling of the linear and the cyclic into one unique
composite movement is the result of a very subtle form of spatial
or temporal hierarchy in which we move perceptually through
a series of steps or stages, much like we might walk from, say,
A to Z.
Remarkably, as we progress through the hierarchy, what we
know to be a straight line mysteriously transforms itself into
a rounded circle, as, once we arrive at Z, Z suddenly changes
into A, bringing us back again to where we started.
In this way, progressing through the steps of a strange loop is
much like descending (or ascending) a kind of surreal, paradoxical
staircase. (Think of the drawings of Dutch graphic artist, Escher, here.)
That is, even though there is a clear hierarchical structure of ordered
steps, the moment we reach the ground floor we unexpectedly, at
the same time, arrive at the top again, and so on, ad infinitum.
Indeed, this is why the loop or cycle is called, 'strange', in that it
generates a perceptual illusion of a kind. At the same time, it is
important to realize that the movement itself is not illusory, but
is indeed very real.
Historically, in the Western musical tradition, two examples of strange
loops come to mind. One is in pitch (or space), and, the other,
in rhythm (or time).
The first can be found in the music of J.S. Bach with The Musical Offering
and the famous Ascending Canon. (Hofstadter calls attention to this
remarkable composition in his book.) The second is a much more recent
piece written by the North American composer, Elliott Carter; it occurs
in the extraordinary central movement of his Double Concerto.
I am deeply indebted to both these composers, especially, in this
context, to Elliott Carter. The Descending Staircase was in part
inspired by some of the rhythmic patterns he discovered back in
the 50's and 60's.
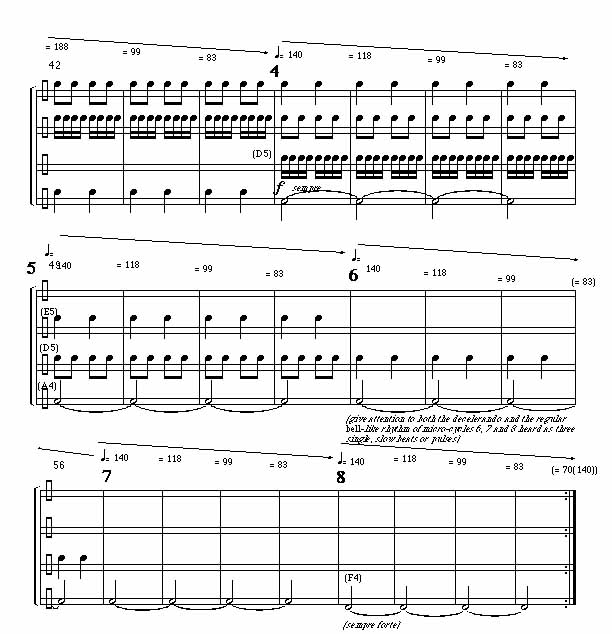
Mathematics and the Shape of Change
As a footnote, for those interested in the relationship between the shape
of change as it is heard and performed in music, and the new mathematics
which might be used to describe it, I've included a little iterative function
which generates the tempo changes used in
the piece:
= 140
The numbers which come out of the equation in reverse --
(approximately 140, 118, 99, 83 and 70) -- create a remarkably
smooth glissando between tempo doublings, or what I call
octaves of tempo: 140 | 70 | 35 | etc. | In The Descending Staircase,
the tempo slides seamlessly through eight such octaves of tempo.
As a closing thought, in Nature, I've always felt that one of the
outstanding examples of a strange loop-like movement can be
experienced directly while pondering the movements of a high
country waterfall, which is, after all, forever ending and beginning
at the same time. A marriage of linear and cyclical, both finite
and infinite indeed!
SEE: (1) CARTER Double CONCERTO, central #strangeloop
movement http://bit.ly/ROPJQG
(2) BACH: ART OF FUGUE: Endlessly Rising
Modulation Canon http://bit.ly/UgqCfI