
m4—for bass clarinet a set of four
frames for virtuoso solo instrument . . .

m4—for bass clarinet a set of
four
frames for virtuoso solo instrument . .
.
| go to scores of I: | 1
| 2 | II: |
1 | 2
| III: | 1 |
2 | IV: |
1 |2
| 3 |
| NEW: Download MP3 of a performance
model of
m4: frames
I-IV [c. 9' 39" 2.1 Mb]
| Listen to a performace model (c. 9' 39") of
m4—version
for bass clarinet in streamed RealAudio |
| NEW: Download PDF of
m4: for solo cello I-IV
(Portable Document File) |
| listen to a m4: frames
I: nightwind;
II: entrance;
III:
dialogue; IV:
sarpa (REQUIRES QuickTime) |
About m4 and frames
Instead of a cycle of movements which characterizes the other Star Cycle compositions
for solo instruments, one might best think of m4 as something more like a set of frames.
Frames are what I think of a 'windows' on, or snap shots off, different qualities of
musical movement. In this regard, frames are rather shorter and more fragmentary
than movements, but at the same time are more suited for exploring a wider diversity
of new ideas. One might think of them literally as a collection of photos from a wild
place which are tossed as it were upon the performance table. We see a new species
of plant here, an outline of a fearsome animal there, even perhaps a hint of free running
white water. So in a way, one can pick up any frame or window one feels attracted to and
set to work.
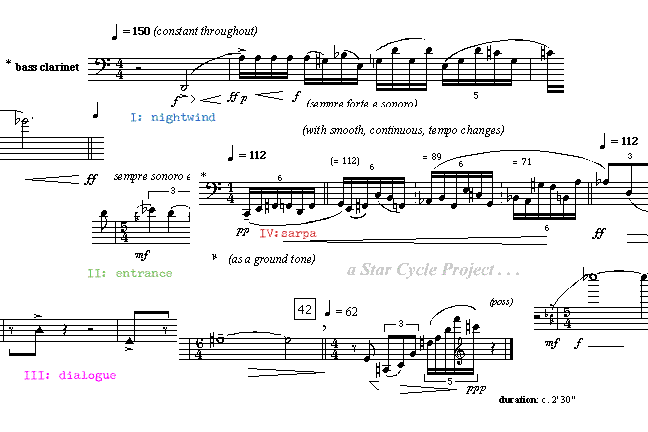
The first frame of m4 featured here introduces the set with a wildly fast and rugged
stream of musical figures which unfolds within a non-stop, forward-driving tempo.
There is a marked contrast between the complexity of what one hears, on the one
hand, and the relative simplicity of the notation on the other. This is because we hear
a stream of serpentine movement, moving in unexpected ways over the entire instrument.
But the notation slows this down, so to speak, visually, by using a neutral 4/4 meter
to structure the whole, which, although it does not change, is very fast. The quarter
note here equals 150 beats per minute. This means that the temporal resolution
the performer must deal with here is very high in deed, as is the density at an
average of about 10 sounds per second (Sps). Besides its simplicity, the great
advantage of this style of notation is that one can begin very slowly and gradually
with practice work on getting the tempo faster and faster while at the same time
not sacrificing any rhythmic precision.
About the rhythmical
ground of change
This first frame of m4 is based on a very general, and, to my way of looking at
musical movement, important concept, ie, that of a rhythmical ground. The basic
idea here is this:
underlying all movement is a kind of implicit, non-manifest, generative
ground which contains within it all possible details of rhythmic pulsation.
Briefly, the sketch below shows all these pulses as we might think of them
(or hear them) going on at the same time.
The dominant stream is the red one, in the middle; it is notated
in groups of four fast, steady pulses.
Above and below this red layer is a stream of 5 and 6 (faster),
and 3 and 2 (slower).
Now, what happens in the course of the music is that the 4-stream from
time to time flips out of its groove and runs a 5 or 6, or slips and steps
into a slower 3 or 2.
The image of movement in the natural world this calls to mind is perhaps
that of running a fast-flowing stream in a raft. We flow along constant
for a while, then encounter eddies and whirlpools, hit rocks,
stretches of slower water, and what not.
Here is what a sketch the rhythmic ground might look like:
And here is a sketch of a single stream emerging from the ground,
pulsing in 4's with one faster group of 5's:
And here is a sketch of a more complex stream, getting faster and slower around
the steady stream of 4's:
And lastly, this is how the above sketch looks after converted into musical notation:
| listen to computer model of m4: frame I: nightwind |
Sarpa: Serpentine tempo slides or glissandi
The fourth frame of m4 is titled Sarpa. (Sanskrit: snake, serpent)
The key musical or rhythmic feature here—the shape of the music's change—
is a smooth, continuous 'getting faster' and 'getting slower'. The music does this
in a necessarily very precise way, moving in steps until the tempo or speed of the
basic meter is doubled, then doubled again, and again. Or vice versa: halved and
halved again, and so forth. This is directly analogous to singing or playing a sliding
tone—a so-called glissando—from one pitch to another one an octave higher, and
so on. That's why I call these doublings of tempo octaves. Here's a sketch of the
cycle of relationships. (Mathematicians, among whom I unfortunately do not include
myself, will notice, to use their language here for a moment, a fractal-like iterative
function at the root of this pattern of movement, with self-similar relationships at
differences of scale. The key remains, however, that it sounds beautiful, much as if
the graceful spirals of ferns had been translated into sound.(see photo/miniature:
metaphor) This phrase—self-similar relationships at a differences of scale—is an
important one to remember, I think. This is because it points to a simple yet powerful
way of looking at or thinking about both structure and movement in the future.):
| To view a sketch of the tempo relationships for Sarpa,
go to Four octaves of tempo |



Copyright © 1999 - 2003 Cliff Crego All Rights Reserved